Tree height
(→Measurement of tree height) |
|||
| (16 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | + | {{Ficontent}} | |
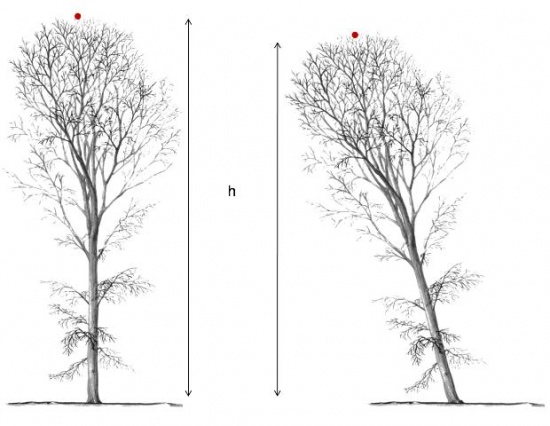
| − | Total tree height is defined to be the perpendicular distance between the ground level and the level of the top of a tree. In contrast tree length is the distance between the stem foot and the top along the stem axis. Obviously tree height and tree length are identical as long as the tree is growing vertically. For oblique trees, the difference between height and length becomes relevant and length is larger than tree height, depending on the inclination angle. | + | Total tree height is defined to be the perpendicular distance between the ground level and the level of the top of a [[Tree Definition|tree]]. In contrast tree length is the distance between the stem foot and the top along the stem axis. Obviously tree height and tree length are identical as long as the tree is growing vertically. For oblique trees, the difference between height and length becomes relevant and length is larger than tree height, depending on the [[angle|inclination angle]]. |
| − | [[file:tree height.jpg|center]] | + | [[file:tree height.jpg|center|550px]] |
| − | Total height is of interest to characterize a stand for example by determining the yield class or to estimate [[ | + | Total height is of interest to characterize a [[Forest Definition|stand]] for example by determining the yield class or to estimate [[Volume functions|tree volume]] or [[Biomass functions and carbon estimation|tree biomass]]. If interest is in commercial volume, it is recommendable to also measure the commercial tree height. This is the height at the stem up to which logs that can be cut are considered as "commercial". The understanding of what is commercial is depending on the wood markets and the conventions used there. Commercial height needs to be defined according to the defined criteria. While there is no general definition, commercial height is mostly defined to stem sections up to the first dead or living branches or the first damages or irregularities affecting wood quality. Further a certain minimum diameter is considered. |
==Measurement of tree height== | ==Measurement of tree height== | ||
| Line 11: | Line 11: | ||
Some general points should be considered for height measurements: | Some general points should be considered for height measurements: | ||
*Tree top and stem base should be visible from the observer's position, | *Tree top and stem base should be visible from the observer's position, | ||
| − | * | + | *distance should be at least equal or larger that tree height, |
| − | * | + | *the inclination of oblique trees has to be considered, |
*multiple measurements of the same tree should not vary more than 0,5 meter, | *multiple measurements of the same tree should not vary more than 0,5 meter, | ||
| − | * | + | *in case of devices using fixed distances (e.g. [[Blume-Leiss]], [[Haga]], [[Suunto]] or Silva [[Clinomaster]]): ensure to use the right scale for the actual distance. |
| + | *as distance is calculated based on the horizontal distance, a correction of tree height is required if the distance is measured as slope distance (modern electronic devices correct automatically)! | ||
==Potential errors in tree height measurements== | ==Potential errors in tree height measurements== | ||
In general errors in the determination of tree heights can have different reasons: | In general errors in the determination of tree heights can have different reasons: | ||
| − | *Error in distance measurement (eventually not the correct [[horizontal distance]]), | + | *Error in distance measurement (eventually not the correct [[Distance to tree|horizontal distance]]), |
*Wrong measurement of angles, | *Wrong measurement of angles, | ||
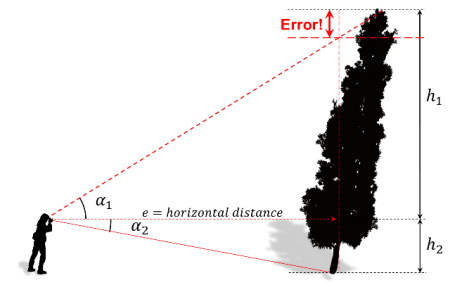
*Tree top is not visible or misinterpreted, | *Tree top is not visible or misinterpreted, | ||
| − | *Misinterpretation of tree height in case of oblique trees. | + | *Misinterpretation of tree height in case of oblique trees, |
| + | *wrong calibration of electronic devices (e.g. [[vertex]]). | ||
{|align="center" | {|align="center" | ||
| − | |[[File: | + | |[[File:tree_height_error1.png|misinterpretation of tree top|450px]] |
| − | |[[File: | + | |[[File:tree_height_error2.png|oblique tree|450px]] |
|} | |} | ||
| − | + | {{SEO|keywords=tree height,forest inventory,tree measurement,single tree variables | |
| − | + | sampling|descrip=Total tree height is defined to be the perpendicular distance between the ground level and the level of the top of the tree.}} | |
| − | + | ||
| − | {{ | + | |
| − | + | ||
[[Category:Tree height]] | [[Category:Tree height]] | ||
Latest revision as of 11:38, 20 April 2016
Total tree height is defined to be the perpendicular distance between the ground level and the level of the top of a tree. In contrast tree length is the distance between the stem foot and the top along the stem axis. Obviously tree height and tree length are identical as long as the tree is growing vertically. For oblique trees, the difference between height and length becomes relevant and length is larger than tree height, depending on the inclination angle.
Total height is of interest to characterize a stand for example by determining the yield class or to estimate tree volume or tree biomass. If interest is in commercial volume, it is recommendable to also measure the commercial tree height. This is the height at the stem up to which logs that can be cut are considered as "commercial". The understanding of what is commercial is depending on the wood markets and the conventions used there. Commercial height needs to be defined according to the defined criteria. While there is no general definition, commercial height is mostly defined to stem sections up to the first dead or living branches or the first damages or irregularities affecting wood quality. Further a certain minimum diameter is considered.
[edit] Measurement of tree height
A direct measurement of tree height (e.g. by means of a pole) is only feasible for relatively small trees and restricted to the assessment of regeneration or younger plantations. More common is the the indirect measurement of tree height based on simple geometric relationships where we distinguish the trigonometric principle and the geometric principle. Many different measurement devices are available, while most of the modern ones calculate tree height based on measured distances and angles.
Some general points should be considered for height measurements:
- Tree top and stem base should be visible from the observer's position,
- distance should be at least equal or larger that tree height,
- the inclination of oblique trees has to be considered,
- multiple measurements of the same tree should not vary more than 0,5 meter,
- in case of devices using fixed distances (e.g. Blume-Leiss, Haga, Suunto or Silva Clinomaster): ensure to use the right scale for the actual distance.
- as distance is calculated based on the horizontal distance, a correction of tree height is required if the distance is measured as slope distance (modern electronic devices correct automatically)!
[edit] Potential errors in tree height measurements
In general errors in the determination of tree heights can have different reasons:
- Error in distance measurement (eventually not the correct horizontal distance),
- Wrong measurement of angles,
- Tree top is not visible or misinterpreted,
- Misinterpretation of tree height in case of oblique trees,
- wrong calibration of electronic devices (e.g. vertex).

|
|