Estimating the length of the forest edge
(→Mapped plots) |
|||
| Line 27: | Line 27: | ||
where ''r'' = radius of plot [m] = 25m, here. | where ''r'' = radius of plot [m] = 25m, here. | ||
| + | |||
The factor 2, in addition to the expansion factor, is introduced in that formula because when sampling only forest plots ‑ that is, plots with their centers inside the forest ‑ one evaluates only approximately half of all sample plots that can potentially intersect with the forest boundary: intersecting sample plots with their centers outside forest are not considered, although they would occur on average in about the same number as the intersecting forest plots. Therefore, assuming symmetry, we double the number of intersecting forest plots – accepting a slight underestimation that comes from the fact that for closed polygons with an overall convex shape, one expects on average several more intersecting circular plots with centers outside that polygon. | The factor 2, in addition to the expansion factor, is introduced in that formula because when sampling only forest plots ‑ that is, plots with their centers inside the forest ‑ one evaluates only approximately half of all sample plots that can potentially intersect with the forest boundary: intersecting sample plots with their centers outside forest are not considered, although they would occur on average in about the same number as the intersecting forest plots. Therefore, assuming symmetry, we double the number of intersecting forest plots – accepting a slight underestimation that comes from the fact that for closed polygons with an overall convex shape, one expects on average several more intersecting circular plots with centers outside that polygon. | ||
| + | |||
Averaging over ''n'' cluster plots yields the estimated mean forest edge length per hectare <math>\hat{L}_{mean}</math> | Averaging over ''n'' cluster plots yields the estimated mean forest edge length per hectare <math>\hat{L}_{mean}</math> | ||
:<math>\hat{L}_{mean}=\frac{\sum_{i=1}^n(l_{ha})_i}{n}\,</math> | :<math>\hat{L}_{mean}=\frac{\sum_{i=1}^n(l_{ha})_i}{n}\,</math> | ||
| − | where <math>(l_{ha}_i=0</math> for those cluster plots that do not intersect. | + | |
| + | where <math>(l_{ha})_i=0</math> for those cluster plots that do not intersect. | ||
The total forest edge length is estimated by expanding to the total area ''A'' [ha] as | The total forest edge length is estimated by expanding to the total area ''A'' [ha] as | ||
:<math>\hat{L}_{tot}=A\frac{\sum_{i=1}^n(l_{ha})_i}{n},\,</math> | :<math>\hat{L}_{tot}=A\frac{\sum_{i=1}^n(l_{ha})_i}{n},\,</math> | ||
| − | |||
and the variance of the estimated total forest edge length is estimated by | and the variance of the estimated total forest edge length is estimated by | ||
| − | :<math>\hat{var}(\hat{L}_{tot}=A^2\frac{\sum_{i=1}^n\left[(l_{ha})_i-\hat{L}_{mean}\right]^2}{n(n-1)}.\,</math> | + | :<math>\hat{var}(\hat{L}_{tot})=A^2\frac{\sum_{i=1}^n\left[(l_{ha})_i-\hat{L}_{mean}\right]^2}{n(n-1)}.\,</math> |
==References== | ==References== | ||
Revision as of 23:18, 16 March 2011
Contents |
General considerations
Forest edge length is an important attribute when quantitatively describing the structure of forested landscapes: for a given forest cover, a longer forest edge usually corresponds to a more fragmented landscape. The forest edge length may also used as indicator for forest fragmentation being relevant for both biodiversity monitoring and the sustainable management of large area forest resources. It is also relevant, for example, for research on the function of forests as a sink for atmospheric deposition: atmospheric deposition of both nutrients and pollutants is higher at forest edges, particularly where the forest edges are exposed to the prevailing wind direction.
For research into and modelling of all three classes of edge effects, the determination of edge lengths in a given study region is required. Even if it is some times stated that, with respect to biodiversity aspects and atmospheric depositions the forest edge structure is more important than forest edge length, the lengths of differently structured forest edges are still relevant input for modelling. Forest edge length determination is frequently done manually or by automatic delineation using remote sensing imagery. However, it can also be done by sample based estimation either in remote sensing imagery or from terrestrial sample plots.
Forest edge length is the length of the virtual line that separates forest from non-forest. Just as it is difficult to clearly define “forest”, it is not simple to clearly define the line where forest ends. It is interesting to note that in most remote sensing based studies the delineation of the forest edge is done without explicitly and clearly giving a “measurement protocol”.
Forest edge length is an important input variable to calculate landscape metrics that describe the spatial pattern of forests; in a comprehensive factor analysis, Riijters et al. (1995[1]) demonstrate that edge length is among the most relevant landscape variables for their derivation. Landscape metrics are usually derived from maps; few studies focus on sample based estimation of these metrics (examples are Hunsaker et al. 1994[2], Kleinn 2000[3]). Rudis (2006[4]) uses sample based forest edge length estimates to characterise forest edge-interior fragmentation. In this article, such sample based estimation approaches for forest edge length are presented. This chapter bases largely on Kleinn and Kändler (in review[5]).
Estimating forest edge length
Based on typical plot designs as used in large area forest inventories, there are various approaches for the estimation of forest edge length. Important in this context is that the spatial resolution of such estimation needs to be considered: the finer the spatial resolution the longer will be the estimated line length. An illustrative example for this intuitive fact is elaborated in Kleinn and Kändler (in review) where forest edge length is estimated from the cluster plot design of the German National Forest Inventory at three different spatial resolutions, resulting in three considerably different results.
There are at least three basic approaches for the estimation of forest edge length differing in the attribute which is observed per plot. They are briefly dealt with in the following sub-chapters.
Mapped plots
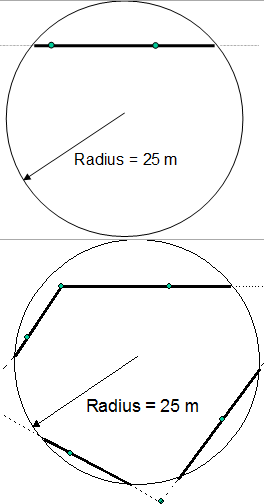
Here, the line length is directly measured on the fixed area plots and estimation follows the usual estimators with plot expansion factors. An example is illustrated in Figure 1 as implemented in the second German National Forest Inventory.
Assuming 25m radius plots, the estimation of forest edge length for an inventory region goes as follows: let \(l_b\) be the observed forest edge length in meters on a 25m radius plot; a per plot estimator of forest edge length per hectare \(l_ha\) results from standard plot expansion by
\[\hat{l}_{ha}=s\frac{10^4}{\pi{r}^2}l_b=\frac{32}{\pi}l_b,\,\]
where r = radius of plot [m] = 25m, here.
The factor 2, in addition to the expansion factor, is introduced in that formula because when sampling only forest plots ‑ that is, plots with their centers inside the forest ‑ one evaluates only approximately half of all sample plots that can potentially intersect with the forest boundary: intersecting sample plots with their centers outside forest are not considered, although they would occur on average in about the same number as the intersecting forest plots. Therefore, assuming symmetry, we double the number of intersecting forest plots – accepting a slight underestimation that comes from the fact that for closed polygons with an overall convex shape, one expects on average several more intersecting circular plots with centers outside that polygon.
Averaging over n cluster plots yields the estimated mean forest edge length per hectare \(\hat{L}_{mean}\)
\[\hat{L}_{mean}=\frac{\sum_{i=1}^n(l_{ha})_i}{n}\,\]
where \((l_{ha})_i=0\) for those cluster plots that do not intersect.
The total forest edge length is estimated by expanding to the total area A [ha] as
\[\hat{L}_{tot}=A\frac{\sum_{i=1}^n(l_{ha})_i}{n},\,\]
and the variance of the estimated total forest edge length is estimated by
\[\hat{var}(\hat{L}_{tot})=A^2\frac{\sum_{i=1}^n\left[(l_{ha})_i-\hat{L}_{mean}\right]^2}{n(n-1)}.\,\]
References
- ↑ Riijters K.H., R.V. O’Neill, C.T. Hunsaker, J.D. Wickham, D.H. Yankee, S.P. Timmins, K.B. Jones and B.L. Jackson. 1995. A factor analysis of landscape pattern and structure metrics. Landscape Ecology, Vol. 10, No. 1, S. 23-39.
- ↑ Hunsaker C.T., R.V. O´Neill, B.L. Jackson, S.P. Timmins, D.A. Levine and D.J. Norton. 1994. Sampling to characterize landscape pattern. Landscape Ecology 9:207-226.
- ↑ Kleinn C. 2000. On large area inventory and assessment of trees outside forests. Unasylva 51:3-10.
- ↑ Rudis V.A. 2006. Characterizing forest edge-interior fragmentation using 0.6-ha ground-based samples with applications to forest resource assessments. The 7th FIA Annual Symposium: 2005 October 3-6, Portland, Maine. Program and Abstracts: 78.
- ↑ 5.0 5.1 Kleinn C. and G. Kändler. Submitted. Estimating forest edge length from large area forest inventory data ‑ the example of the German National Forest Inventory. Submitted to CJFR.