Stem volume
(→Volume calculation by section) |
(→The taper curve) |
||
| Line 38: | Line 38: | ||
===The taper curve=== | ===The taper curve=== | ||
| + | |||
| + | The taper curve gives stem radii or [[Tree diameter|diameters]] as a function of [[:Category:Tree height|height]]. It is assumed that the stem has a perfectly circular shape so that only one side of the curve needs to be determined. Once the taper curve is known mathematically, the stem volume can easily be calculated by a rotation integral of this curve. Of course, this is not only possible for the entire stem, but also for any stem section in which we might be interested. This makes the taper curve a very useful tool, for example for assortment purposes. Figure 3 illustrates the concept of a taper curve. | ||
| + | The complete taper curve goes from the bottom of the stem (usually the felling cut) up to the top of the tree where radius is 0. | ||
| + | |||
| + | When a taper curve is to be determined for a particular tree, a number of diameter measurements have to be taken at different heights. And it is a matter of cost-efficiency to try to define the heights where the diameters are to be measured such that as few as possible measurements need to be taken in order to achieve a defined precision. | ||
==References== | ==References== | ||
Revision as of 17:32, 18 February 2011
| sorry: |
This section is still under construction! This article was last modified on 02/18/2011. If you have comments please use the Discussion page or contribute to the article! |
Contents |
General observations
When thinking in terms of timber-production oriented forest management, stem volume is one of the most relevant variables in forest inventory. The volume unit in the metric system is cubic meter (\(m^3\)). Stem volume is one of those variables that can not be measured directly in a forest inventory but that needs to be modeled and predicted from more easily measurable variables. Volume is either determined for the entire stem (total volume) or for defined stem sections (such as commercial volume).
Calculating volume
Direct measurement
The only method of tree volume measurement is by water displacement. However, this is a theoretical approach and not an option in forest inventory…
Volume calculation by section
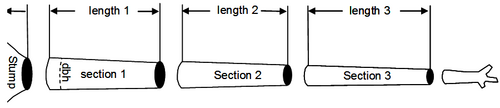
Tree volume determination is always based on measurements of diameter and length. When we do a section-wise volume calculation, the stem is subdivided into sections (Figure 1) of which length \(l\) and basal area \(g\) are measured; basal area is either taken at the middle of the section \(g_m\) or at both the lower end \(g_l\) and the upper end \(g_u\). Then, the following simple formulae are common for section volume calculation:
Huber \(v=g_ml\,\) Smalian \(v=\frac{g_u+g_l}{2}l\,\) Newton \(v=\frac{g_u+4g_m+g_l}{6}l\,\)
where the “Huber formula” is simply another name for the volume formula of a cylinder.
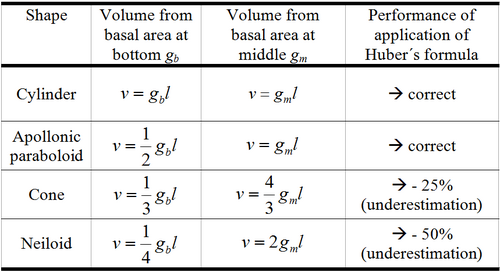
All three formulae give the correct result if a section has a quadratic paraboloidal shape or is a cylinder. Newton's formula also applies for a section of a cone. If the section or a log is neither a quadratic paraboloid nor a cylinder, then the Smalian’s or Huber’s formula will produce relatively more errors. For a conoid, i.e. a section of a cone, Smalian's formula overestimates the volume of a section, whereas Huber's formula underestimates the volume by half the amount of the overestimation with Smalian's. The errors given by both formulae are proportional to the length of the log and the square of the difference between the diameters at the two ends. Volumes of logs are normally estimated employing Huber's formula using logs that are as short as is practical.
There are four basic geometric solids with which stem sections can usually be modeled; that is: the cylinder, the apollonic paraboloid, the cone and the neiloid (Figure 2).
The taper curve
The taper curve gives stem radii or diameters as a function of height. It is assumed that the stem has a perfectly circular shape so that only one side of the curve needs to be determined. Once the taper curve is known mathematically, the stem volume can easily be calculated by a rotation integral of this curve. Of course, this is not only possible for the entire stem, but also for any stem section in which we might be interested. This makes the taper curve a very useful tool, for example for assortment purposes. Figure 3 illustrates the concept of a taper curve. The complete taper curve goes from the bottom of the stem (usually the felling cut) up to the top of the tree where radius is 0.
When a taper curve is to be determined for a particular tree, a number of diameter measurements have to be taken at different heights. And it is a matter of cost-efficiency to try to define the heights where the diameters are to be measured such that as few as possible measurements need to be taken in order to achieve a defined precision.
References
- ↑ Kleinn, C. 2007. Lecture Notes for the Teaching Module Forest Inventory. Department of Forest Inventory and Remote Sensing. Faculty of Forest Science and Forest Ecology, Georg-August-Universität Göttingen. 164 S.