Accuracy and precision
| Line 4: | Line 4: | ||
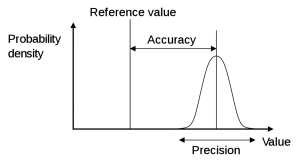
accuracy of estimates is the degree of "closeness" to the actual (true) value, while the precision of estimates is the degree to which repeated estimates under unchanged conditions show the same results. A simple definition of “accuracy” is therefore the freedom from mistake or error: '''correctness'''. While “precision” is defined as the quality or state of being precise: '''exactness'''. | accuracy of estimates is the degree of "closeness" to the actual (true) value, while the precision of estimates is the degree to which repeated estimates under unchanged conditions show the same results. A simple definition of “accuracy” is therefore the freedom from mistake or error: '''correctness'''. While “precision” is defined as the quality or state of being precise: '''exactness'''. | ||
| − | [[file:accuracy.png|thumb|left|Accuracy and precision]] | + | [[file:accuracy.png|thumb|left|300px|Accuracy and precision]] |
| Line 10: | Line 10: | ||
|message=Example: | |message=Example: | ||
|text='''Accuracy:''' | |text='''Accuracy:''' | ||
| − | Imagine you have the task to assess the mean height of students in a class. Unfortunately all you have for measuring the height is an old ruler, that has gotten a little longer with time. Thus, a [[bias]] is introduced by systematically overestimating the real height of each student. As a result the mean height for the whole class will also be too high. Here we say: the accuracy of mean height is low (even if the precision of estimates can be high). | + | Imagine you have the task to assess the mean height of students in a class. Unfortunately all you have for measuring the height is an old ruler, that has gotten a little longer with time. Thus, a [[bias]] is introduced by systematically overestimating the real height of each student. As a result the mean height for the whole class will also be too high. Here we say: the accuracy of mean height is low (even if the precision of estimates can be high). |
| + | |||
| + | '''Precision:''' | ||
| + | Using a ruler (which varies its length due tobad manufacturing), you want to measure your own height. Whilemeasuring your height several times in a row, you recognize that youmeasure a different height with every measurement. The variation ofeach measurement around the mean height (your real height) gives you anidea about the precision of the measurement. | ||
}} | }} | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
[[Category:Introduction to sampling]] | [[Category:Introduction to sampling]] | ||
Revision as of 14:12, 15 November 2010
Accuracy and precision are two terms that are often equivalently used, even though they do not have the same meaning and should be used accordingly. The accuracy of estimates is the degree of "closeness" to the actual (true) value, while the precision of estimates is the degree to which repeated estimates under unchanged conditions show the same results. A simple definition of “accuracy” is therefore the freedom from mistake or error: correctness. While “precision” is defined as the quality or state of being precise: exactness.
Imagine you have the task to assess the mean height of students in a class. Unfortunately all you have for measuring the height is an old ruler, that has gotten a little longer with time. Thus, a bias is introduced by systematically overestimating the real height of each student. As a result the mean height for the whole class will also be too high. Here we say: the accuracy of mean height is low (even if the precision of estimates can be high).
Precision: Using a ruler (which varies its length due tobad manufacturing), you want to measure your own height. Whilemeasuring your height several times in a row, you recognize that youmeasure a different height with every measurement. The variation ofeach measurement around the mean height (your real height) gives you anidea about the precision of the measurement.