The trigonometric principle
From AWF-Wiki
(Difference between revisions)
(→Basic principle) |
|||
| Line 2: | Line 2: | ||
=Basic principle= | =Basic principle= | ||
| − | The so-called trigonometric principle of | + | The so-called trigonometric principle of height measurement bases on the measurement of angles as illustrated in the following figure. Height values per inclination angle are then calculated by |
| + | |||
| + | :<math>h=e*\tan \alpha \,</math>, | ||
| + | |||
| + | if the inclination is measured in degrees or | ||
| + | |||
| + | :<math>h=e*\frac{p}{100} \,</math>, | ||
| + | |||
| + | if inclination is measured in percent; ''e'' is the [[horizontal distance]]. These basic formula allow calculating what is given as ''h1'' and ''h2'' in the figure. | ||
[[file:trigonometric_principle.jpg|center]] | [[file:trigonometric_principle.jpg|center]] | ||
Revision as of 08:02, 4 November 2010
 |
sorry: |
This section is still under construction! This article was last modified on 11/4/2010. If you have comments please use the Discussion page or contribute to the article! |
Basic principle
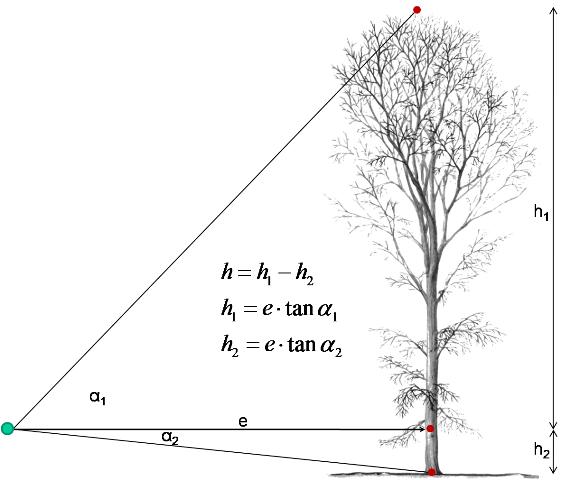
The so-called trigonometric principle of height measurement bases on the measurement of angles as illustrated in the following figure. Height values per inclination angle are then calculated by
\[h=e*\tan \alpha \,\],
if the inclination is measured in degrees or
\[h=e*\frac{p}{100} \,\],
if inclination is measured in percent; e is the horizontal distance. These basic formula allow calculating what is given as h1 and h2 in the figure.
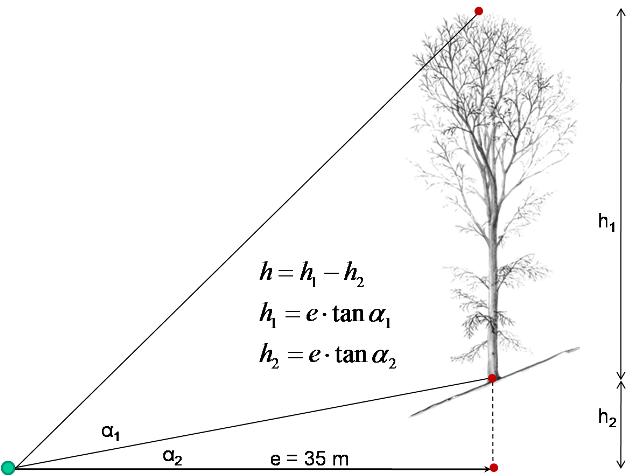
Measuring tree heights in sloped terrain
Note:
- If one uses a measurement device which does not enable to directly measure horizontal distances (or e.g. rely on fixed distances to the tree determined by optical principles) the measured distance has to be corrected. To avoid corrections in sloped terrain a practical solution is to move parallel to the slope and to find a position from which one can directly measure the horizontal distance.
Measuring height without given distance
